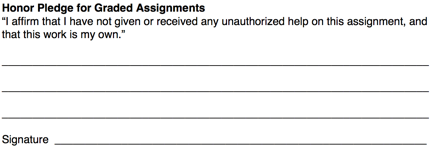
Write the following text on the front cover of your homework assignment and
sign it. If the text is missing, 20 points will be subtracted from your
homework grade.
1. Consider the line connecting (x1, y1) = (0, 0) and (x2, y2) = (1, 1). Show explicitly that the function y(x) = x produces a minimum path length by using the varied function y(a, x) = x + a sin{p(1 - x)}. Use the first few terms in the expansion of the resulting elliptic integral to show the equivalent of Equation 6.4 in the textbook.
2. A disk of radius R rolls without slipping inside the parabola y = ax2. Find the equation of constraint. Express the condition that allows the disk to roll so that it contacts the parabola at one and only one point, independent of its position.
3. a. Find the curve y(x) that passes through the endpoints (0, 0) and (1, 1) and minimizes the functional
![]()
b. What is the minimum value of the integral?
c. Evaluate I[y] for a straight line y = x between the points (0, 0) and (1, 1).
4. The corners of a rectangle lie on the ellipse (x/a)2 + (y/b)2 = 1.
a. Where should the corners be located in order to maximize the area of the rectangle?
b. What fraction of the area of the ellipse is covered by the rectangle with maximum area?
This set covers the material discussed in Chapter 6.