In this Section we will introduce the concepts of work and kinetic energy.

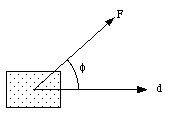
Figure 1. A force F acting on a body. The resulting displacement is indicated by the vector d.
Suppose a constant force F acts on a body while the object moves over a distance d. Both the force F and the displacement d are vectors who are not necessarily pointing in the same direction (see Figure 7.1). The work done by the force F on the object as it undergoes a displacement d is defined as
![]()
The unit of work is the Joule (J). The work done by the force F is zero if:
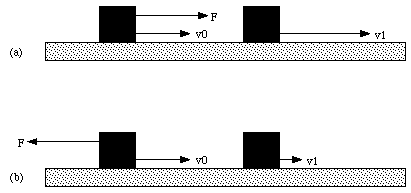
Figure 2. Positive or Negative Work.
The work done by the force F can be positive or negative, depending on [phi]. For example, suppose we have an object moving with constant velocity. At time t = 0 s, a force F is applied. If F is the only force acting on the body, the object will either increase or decrease its speed depending on whether or not the velocity v and the force F are pointing in the same direction (see Figure 7.2). If the force and the velocity of the object are pointing in the same direction, the speed of the object will increase and the work done by the force on the object will positive. If the force and the velocity of the object are pointing in the opposite direction, the speed of the object will decrease and the work done by the force on the object will be negative.
The observation that an object is moving with a certain velocity indicates that at some time in the past work must have been done on it. It turns out that the work done by the force is equal to the change in a quantity called the kinetic energy K. The kinetic energy K is defined as
![]()
where m is the mass of the object and v is its velocity. The unit of kinetic energy is the Joule (J).
If the kinetic energy of a particle changes from some initial value Ki to some final value Kf the amount of work done on the particle is given by
W = Kf - Ki
This indicates that the change in the kinetic energy of a particle is equal to the total work done on that particle by all the forces that act on it. The relation between work and kinetic energy is called the work-energy theorem. This is a very useful relation and is often used to determine the forces required to produce a change in motion. Consider for example a collision. The initial kinetic energy of a car involved in the collision is
Ki = (1/2) m vi^2
The final kinetic energy of the car, assuming is comes to rest after the collision (vf = 0 m/s), is
Kf = (1/2) m vf^2 = 0 J
Thus the work required to bring the car to rest is equal to
W = Kf - Ki = (1/2) m vi^2
The work done during the collision is the work done by collision force Fcol:
W = Fcol d = (1/2) m vi^2
The collision force is thus equal to
Fcol = (m vi^2) / (2 d)
We conclude several things: