CHAPTER 1

UNITS

1. UNITS

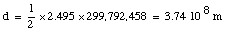
Measurements of physical quantities take place by means of a comparison with a standard. For example: a meter stick, a weight of 1 kilogram, etc.

The base units that will be used in this course are:

meter (m): One meter is equal to the path length traveled by light in vacuum during a time interval of 1/299,792,458 of a second.
kilogram (kg): One kilogram is the mass of a Platinum-Iridium cylinder kept at the International Bureau of Weights and Measures in Paris.
second (s): One second is the time occupied by 9,192,631,770 vibrations of the light (of a specified wavelength) emitted by a Cesium-133 atom.

A unit that is being used as a base unit must be both accessible and invariable. The original meter bar kept in Paris was not very accessible (this is still true for the kilogram). In addition, the length of the standard bar is temperature dependent. The definition of the meter in terms of the number of wavelengths of a particular atomic transition in Krypton-86 made the meter more accessible; the transition is characteristic for Krypton-86, and is the same for each Krypton-86 atom. However, Doppler shifts due to the thermal motion of the atoms can slightly change the wavelength, and produce a small uncertainty in the definition of the meter. The current definition of the meter in terms of the speed of light is not affected by thermal motion: the speed of light in vacuum is constant, independent of temperature and velocity of observer and/or source.

Example: how to measure the distance d from the earth to the moon ?

APOLLO astronauts placed a mirror on the moon. It can be used to measure the distance between the earth and the moon very accurately. The reflection of a laser beam aimed at this mirror reaches the earth after 2.495 s. The distance can then be calculated:

The definition of the standard mass makes it very inaccessible. In principle, the weight of individual nuclei can be used as a standard; nuclear weight does not depend on location, temperature, pressure, etc. However, counting the number of nuclei in a standard (or assembling a fixed number of nuclei) is an almost impossible task.

Note:

1. Improved definitions of base units must be defined such that it matches the previous definition as closely as possible (no need to change all meter sticks in 1983).

2. Speed of light is now defined as 299,792,458 m/s.

All physical quantities are expressed in terms of base units. For example, the velocity is usually given in units of m/s. All other units are derived units and may be expressed as a combination of base units. For example (see Appendix A):

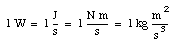
Other examples are:

density: kg/m³
area: m²

When dealing with very small or large numbers, it is convenient to use prefixes (see Table 1.1, and Table 1-2 on page 3 in Halliday, Resnick, and Walker).

Factor	Prefix	Symbol
10¹⁸	exa-	E
10¹⁵	peta-	P
10¹²	tera	T
10⁹	giga-	G
10⁶	mega-	M
10³	kilo-	k
10^-3	milli-	m
10^-6	micro-	u
10^-9	nano-	n
10^-12	pico-	p
10^-15	femto-	f
10^-18	atto-	a

Table 1.1. SI Prefixes

Example: 2.35 10^-9 s = 2.35 ns

In many cases, the data are not supplied in the correct units, and one needs to convert units (see Appendix F in Halliday, Resnick, and Walker).

Examples: 1" = 2.54 x 10^-2 m

55 miles = 88 x 10³ m

1 h = 3600 s

55 miles/h = 88 x 10³/3600 m/s = 24.4 m/s

Send comments, questions and/or suggestions via email to wolfs@pas.rochester.edu and/or visit the home page of Frank Wolfs.