1. The position of your car during a recent road trip on
the interstate highway (essentially a straight line) is described by the
position-time graph below, where North is assigned to be positive. You should begin this problem by
redrawing the graph on a whiteboard or blank sheet of paper. Draw it large!
(a) When is the car’s speed zero?
(b) Determine the car’s approximate average velocity for the intervals
(i)
from 0 to 6 hrs
(ii)
from 2 to 4 hrs
(iii)
from 4 to 11 hrs
(c) Determine the car's average speed for its entire 11-hour motion.
(d)
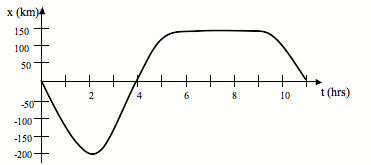
Sketch the velocity versus time graph
corresponding to this motion.
(e) From the graph below, estimate the average
acceleration in the interval from 1 to 3 hours.
(f) At what times is the magnitude of the acceleration
large? When is it positive? When
is it negative?
2.
Smoky the cat is
relaxing on the arm of a couch, one meter above the ground, when he is startled
by something and jumps straight up in the air with initial speed 4 m/s. Coming down, he misses the couch and
lands on the ground. You can
neglect air resistance in your answers below.
(a)
What is Smoky’s acceleration…
(i)
…just after his paws
leave the couch and he is on his way up?
(ii)
…at the exact instant
when he is at his maximum height?
(iii)
…just before he hits the
ground on his way back down?
(b) What is Smoky’s maximum height above the ground during
his motion?
(c)
What is Smoky’s velocity
just before he hits the ground?
(d) How long is Smoky in the air?
3.
You
are on the roof of the lecture hall, 50 m above the ground. As your physics
professor, who is 1.8 m tall, walks toward the hall at a constant speed of 1.20
m/s. If you wish to drop an egg on your professor's head (and commit P121
suicide), where should the professor be when you release the egg? Assume the
egg is in free fall (i.e., you can ignore air resistance). (Actual
experimentation is discouraged.)
4.
A
player kicks a football at an angle of 40 degrees above the horizontal with an
initial speed of 14 m/s. Air
resistance may be ignored. A
second player standing at a distance of 26 m from the first (in the direction
of the kick) starts running to meet the ball at the instant it is kicked. How fast must the second player run in
order to catch the ball just before it hits the ground? How would this answer change if the
football game took place on the moon? (Assume "g" on the moon is 1/6
that on the surface of the earth.
Can you calculate that on your own?)
5.
A
moving sidewalk in an airport terminal building moves at 1.0 m/s and is 40.0 m
long. If a woman steps on at one
end and walks at 2.0 m/s relative to the moving sidewalk, how much time does
she require to reach the opposite end if she walks a) in the same direction the
sidewalk is moving? b) in the
opposite direction? Suppose this
is an escalator instead of a moving sidewalk. Suppose the escalator rises at an angle of 30 degrees with
the floor. Suppose a woman walks
up the escalator with a speed of 2 m/s, what is her horizontal speed with
respect to the floor? What is her
vertical speed with respect to the floor?
What is her total speed with respect to the floor?