Physics 121, Midterm Exam #3

Tuesday April 20, 2004

8.00 am – 9.30 am

 

Do not turn the pages of the exam until you are instructed to do so.

You are responsible for reading the following rules carefully before beginning.

 

Exam rules: You may use only a writing instrument while taking this test.  You may not consult any calculators, computers, books, notes, or each other.

 

Procedure: Answer the multiple-choice questions (problems 1 – 10) by marking your answer on the scantron form.  For each multiple-choice question (problems 1 – 10), select only one answer.  Questions with more than one answer selected will be considered incorrect.  Problems 11, 12, and 13 must be answered in the blue exam booklet and need to be well motivated and expressed in terms of the variables used in the problem.  You will receive partial credit where appropriate, but only when we can read your solution.  Answers that are not motivated will not receive any credit, even if correct.

At the end of the exam, you must hand in the blue exam booklet and the scantron form.  All items must be clearly labeled with your name and student ID number.  If any of these items are missing, we will not grade your exam, and you will receive a score of 0 points.

 

Note:  You are not allowed to use a cheat sheet on this exam.  Please refer to the formula sheet at the end of this package for important equations.

Note:  If you do not answer a question in terms of the variables provided, you will not receive credit for that question.

Note:  You will get 2.5 extra points if you put your student ID correctly on your scantron form.  If you skip a line or forget to fill in the appropriate circles you will not receive these extra points.

Problem 1 (2.5 points)

Which of the following is not a vector?

c   Angular velocity.

c   Angular acceleration.

c   Angle.

c   Torque.

 

Problem 2 (2.5 points)

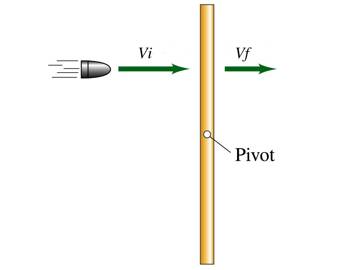
As you hold the string, a yoyo is released from rest so that gravity pulls it down, unwinding the string.  What is the angular acceleration of the yoyo, in terms of the string radius R, the moment of inertia I, and the mass M?

c   g/(R + 2I/(MR))

c   gMR/I

c   gMR/(I + MR2)

c   g/R

 

Problem 3 (2.5 points)

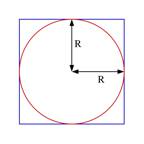
The moment of inertia of a square plate of area 4R2 and mass M, with respect to an axis through its center and perpendicular to the plate, is equal to (2/3)MR2.  A disk of radius R is removed from the center of the plate (see Figure).  What is the moment of inertia of the remaining material with respect to the same axis?

AppleMark

 

c    (p/8)MR2

c   (1/3 - p/12)MR2

c   (1/6)MR2

c   (2/3 - p/8)MR2

 

Problem 4 (2.5 points)

Stcm = dLcm/dt

c   only if the center of mass is at rest

c   only if the center of mass is not accelerating

c   even if the center of mass is accelerating

c   even if the center of mass is accelerating, provided the torque is constant

 

Problem 5 (2.5 points)

The precession rate of the a spinning top

c   is proportional to its angular momentum

c   does not depend upon its angular momentum

c   is inversely proportional to its angular momentum

c   is inversely proportional to its kinetic energy

 

Problem 6 (2.5 points)

Which of the following is the greatest for concrete?

c   tensile strength

c   compressive strength

c   shear strength

 

Problem 7 (2.5 points)

An object will return to its original length if the applied force is removed, provided it has not exceeded its

c   proportionality limit

c   breaking point

c   elastic modulus

c   elastic limit

 

Problem 8 (2.5 points)

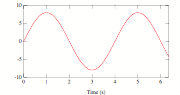
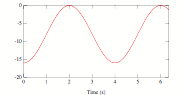
Consider the following graph, showing position versus time for simple harmonic motion. 

 

 

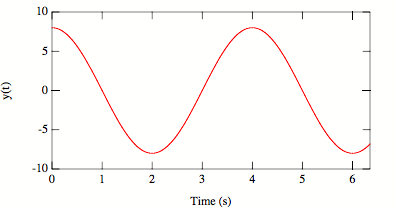
What is the frequency of this motion?

c   0.25 Hz

c   0.50 Hz

c   1.0 Hz

c   4.0 Hz

 

Problem 9 (2.5 points)

Consider simple harmonic motion with amplitude A.  At what displacement in x is the energy shared equally between kinetic energy and potential energy?

c   A

c   A/2

c   Ã2 A

c   A/Ã2

 

Problem 10 (2.5 points)

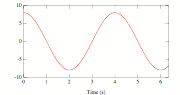
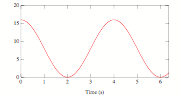
Which of the following graphs could represent the kinetic energy of simple harmonic motion as function of time?

A

 

B

 

C

D

 

c   A

c   B

c   C

c   D

 

Problem 11 (25 points)

A block of mass m, resting on a frictionless surface, is connected to two springs with spring constants k1 and k2.

a.     Consider the configuration shown in the following figure.  Both springs are in their equilibrium (un-stretched) position.

 

 

The block is now moved a distance x to the right.  What is the force that is exerted by the springs on the block (specify direction and magnitude) if the block is held at this position?

b.     When the block is released, it will carry out simple harmonic motion.  What is the period of the motion of the block?

c.     Consider the configuration shown in the following figure.  Both springs are in their equilibrium (un-stretched) position.

 

 

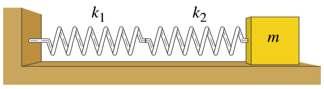
The block is now moved a distance x to the right.  What is the force that is exerted by the springs on the block (specify direction and magnitude) if the block is held at this position?

d.     When the block is released, it will carry out simple harmonic motion.  What is the period of the motion of the block?

Express all your answers in terms of k1, k2, and m.

 

Problem 12 (25 points)

Consider a trailer of mass M connected to a truck, as shown in the Figure below.  The truck and trailer are at rest on a horizontal surface.  The hitch at B can be modeled as a pin support.

 

 

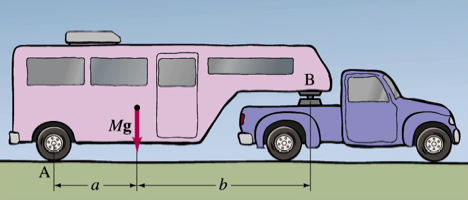
a.     Draw a free-body diagram of the trailer.  Be sure to include all forces acting on the trailer.

b.     What is the force exerted by the road on the rear tires?

c.     What is the force exerted on the trailer by the pin support at B?

Express your answers in term of M, a, and b.

Problem 13 (25 points)

A uniform stick of length H and mass M, initially at rest, is pivoted at its center.  A bullet of mass m is shot through the stick, midway between its pivot and one end (see Figure).

 

 

The bullet approaches the stick with a velocity vi and leaves with a velocity vf = (1/2)vi.  You can ignore the change in the mass and the moment of inertia of the rod as a result of the bullet passing through it.  You can also ignore the effect of gravity.

a.     What is the initial angular momentum of the bullet with respect to the pivot point?  Specify both its magnitude and its direction.

b.     What is the final angular momentum of the bullet with respect to the pivot point?  Specify both its magnitude and its direction.

c.     With what angular speed is the stick spinning after the collision?

d.     How much energy is lost in the collision?

Express all your answers in terms of m, M, H, and vi.