Problem 1
A ball is dropped from the edge of a cliff. Soon after, a second ball is dropped. As a function of time the separation between the two balls
c stays the same.
g increases.
c decreases.
c it depends on the time specified.
Problem 2
Which of the following statements is true for two balls thrown in the air with the same speed at different angle with the horizontal? Ignore air friction.
g The ball making the steeper angle spends more time in the air.
c The ball making the shallower angle spends more time in the air.
c The time of flight depends only on the initial speed given to each ball.
Problem 3
Two balls are projected off a cliff. One is thrown horizontally while the other is released from rest and falls vertically. Which of the following statements is true?
c The ball that falls vertically hits the ground first.
c The ball that is projected horizontally hits the ground first.
g Both balls hit the ground at the same time.
c We can not determine which ball hits the ground first unless we know the speed at which the first ball was projected horizontally.
Problem 4
An elevator has a frayed cable which will break if the tension exceeds a certain value. The tension more likely to exceed this value if the elevator is
c moving at constant velocity
g accelerating upward
c accelerating downward
c the motion is irrelevant
Problem 5
Two blocks of the same size but different masses, m1 and m2, are placed on a table side-by-side in contact with each other. Assume that m1 > m2. Let N1 be the normal force between the two blocks when you push horizontally on the free side of m1 (towards m2). Let N2 be the normal force between the two blocks when you push horizontally on the free side of m2 (towards m1). Which of the following statements is true?
c N1 = N2
g N1 < N2
c N1 > N2
Problem 6
A horizontal force measured with a spring scale is applied to a box sitting on a table. Until the force is increased to a particular value the box does not move. Just as the box starts moving the reading on the spring scale
c remains the same.
g decreases.
c increases.
c more information is needed.
Problem 7
A skier accelerates down a slope inclined at an angle q. From this information we conclude that
c mk > tanq
g mk < tanq
c mk = tanq
c mk = ms
Problem 8
A stunt car goes around a loop-the-loop, hanging upside down at the top. The car does not fall because
g there is a downward force on the car
c there is an upward force on the car
c there is a sideways force on the car
Problem 9
A ball slides down an inclined track and then rounds a loop-the-loop. The ball is released from an initial height so that it has just enough speed to go around the loop without falling off. At the top of the loop-the-loop the normal force of the loop on the ball is
c equal to the weight of the ball and pointing down.
c equal to the weight of the ball and pointing up.
c equal to twice the weight of the ball and point up.
g equal to zero.
Problem 10
The time it takes a falling object to attain its terminal velocity
g increases with increasing mass
c decreases with increasing mass
c is independent of mass
Problem 11
a. The total external force acting on the system is F. The total mass of the system is M1 + M2 + M3. Since the problem states that mass M1 does not move with respect to mass M3, we conclude that all masses are at rest with respect to each other. The acceleration of the system is thus equal to
Since all masses are at rest with respect to each other, the acceleration of mass M3 will be the same as the acceleration of the system. Thus
![]()
b. Since mass M1 does not move with respect to mass M3, the acceleration of mass M1 will be the same as the acceleration of mass M3 (which was calculated in part a). The net force on M1 must thus be equal to
![]()
c. The only force acting on mass M1 in the horizontal direction is the tension in the string which has a magnitude equal to the gravitational force acting on mass M2. This force has a magnitude of M2g. In order for mass M1 does not move with respect to mass M3, the net force calculated in part b must be equal to M2g. We thus require that
![]()
or
![]()
d. If the magnitude of the external force F is less than the magnitude calculated in part c, mass M1 will move forward with respect to the position of mass M3 (consider for example what happens when the external force is 0). If the magnitude of the external force F is more than the magnitude calculated in part c, mass M1 will move backward with respect to the position of mass M3
Problem 12
a. Since the sphere carries out circular motion there must be a net force acting on it in the horizontal plane, directed towards the center of the circle, with a magnitude equal to
![]()
where Lcosq is the radius of the circle.
b. Since the position of the sphere in the vertical direction does not change, the acceleration in the vertical direction is zero, and the net force must be zero.
c. The only force that can provide the required horizontal force is the tension T in the string. The horizontal component of the tension T is equal to
![]()
Combining this with the answer of part (a) we obtain the following expression for the tension T:
![]()
The vertical component of the tension T is equal to
![]()
In order for the net force in the vertical direction to be equal to 0, the magnitude of the vertical component of the tension T must be equal to the gravitational force acting on the sphere:
![]()
The tension T must thus be equal to
![]()
d. Combing the two expressions for T obtained in (c) we conclude that
![]()
or
![]()
From this expression we see that if v increases, cosq must increase and sinq must decrease. This will happen when q decreases. We also see that the relation between speed v and angle q is independent of the mass of the sphere.
e. When the string breaks, the vertical motion of the sphere can be described in terms of motion with a constant acceleration in the vertical direction with an acceleration equal to -g with an initial velocity of 0 m/s. The equation of motion for the position in the vertical direction, assuming the origin is located at the position of the sphere at the moment the string breaks, is given by
![]()
The time that the sphere reaches the ground requires that
![]()
or
![]()
Problem 13
a. Consider the free-body diagram shown in the Figure below.
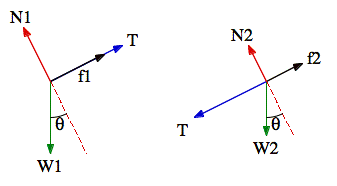
Since the masses move along the ramp, there is no motion in the direction perpendicular to the ramp. The net force in this direction must thus be equal to 0. For block 1 we thus require that
![]()
b. The same arguments use to calculate the normal force on block 1 can be used to calculate the normal force on block 2. For block 2 we fins that
![]()
c. First consider the entire system (2 blocks and string). This system moves down the ram with an acceleration a. The net force required to generate this acceleration is equal to
![]()
The acceleration of the system will thus be equal to
![]()
All components of this system have the same acceleration, and the acceleration of block 1 is thus equal to
![]()
d. The net force on block 1 is equal to the product of its acceleration and its mass:
![]()
The net force can also be expressed in terms of the weight, the friction force, and tension T:
![]()
Comparing these two expressions for F1 we conclude that
![]()
or
![]()