Experiment P03:

Instantaneous Speed versus Average Speed

Concept: linear motion

Time: 30 m

SW Interface: 700

Macintosh® file: P03 Average Speed

Windows® file: P03_AVSP.SWS

EQUIPMENT NEEDED

 

PURPOSE

In this laboratory activity you'll investigate the relationship between instantaneous speed and average speed, and see how a series of average speeds can be used to deduce an instantaneous speed.

 

THEORY

An average speed can be a useful value. It's the ratio of the overall distance an object travels and the amount of time that the object travels. If you know you will average 50 miles per hour on a 200 mile trip, it's easy to determine how long the trip will take. On the other hand, the highway patrol office following you doesn't care about your average speed over 200 miles. The patrol officer wants to know how fast you're driving at the instant the radar strikes your car, so he or she can determine whether or not to give you a ticket. The officer wants to know your instantaneous speed.

If you measure average speed of a moving object over smaller and smaller intervals of distance, the value of the average speed approaches the value of the object's instantaneous speed.

 

PROCEDURE

In this activity, you will use two photogates to measure the amount of time it takes for an object to move a measured distance. You will enter the measured distance into the computer. The Science Workshop program will calculate the average speed based on the distance you enter, and the time that is measured. Then you will change the distance over which the object's motion is measured and repeat the process.

 

PART I: Computer Setup

 

PART II: Sensor Calibration and Equipment Setup

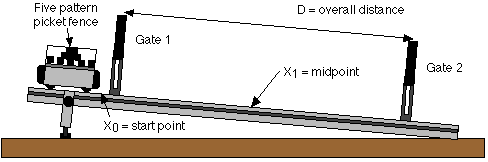
  1. You do not need to calibrate the photogate.
  2. Place the dynamics track on a table. Raise one end of the track several centimeters.
  3. Mark a point at the center of the track with a pencil, and record the point as "X1" in the Data Table.
  4. Choose another point near the top end of the track as the starting point for the dynamics cart, and mark the point with a pencil as "X0".
  5. Place the photogate connected to Digital Channel 1 ("Gate 1") at the top end of the track 40 cm away from the X1 point. Place the photogate connected to Digital Channel 2 ("Gate 2") at the bottom end of the track 40 cm away from the X1 point.
  6. Place the "five-pattern picket fence" into the accessory tray on the top of the dynamics cart. Place the picket fence so that one of the solid bands will block the photogate beam as the cart moves down the track.
  7. Put the cart on track. Adjust the height of both photogates so that the photogate beams are blocked when the cart and picket fence move down the track.

 

Preparing to Record Data

  1. Before recording any data for later analysis, you should experiment with the photogate, cart, and picket fence. Put the cart at the starting point on the track
  2. Click the "REC" button in the Experiment Setup window. The "Keyboard Sampling" window opens.
  3. Release the cart so it moves down the track. Data recording begins when the photogate beam is first blocked.
  4. After the cart moves through the second photogate, type in the distance between the photogates as "Entry #1". For this example, type in "0.80" (80 centimeters or 0.80 meters). Click "Enter".
  5. Click "Stop Sampling" to end recording of your sample data. "Run #1" will appear in the Experiment Setup window.
  6. Click the "Autoscale" button in the Graph display.
  7. Erase your trial run of data. Select "Run #1" in the Data list in the Experiment Setup window......and press the "Delete" key.

 

PART III: Data Recording

  1. Measure the overall distance "D" between the centers of the two photogates. Record the value of D in the Data Table.
  2. Place the cart at the starting point on the track.
  3. Click the "REC" button in the Experiment Setup window. The "Keyboard Sampling" window opens.
  4. Release the cart so it moves down the track. Data recording begins when the photogate beam is first blocked.
  5. After the cart moves through the second photogate, type in the distance between the photogates as "Entry #1". (For example, 80 centimeters or 0.80 meters.)
  6. Click "Enter" to record your value of distance.
  7. Move the two photogates closer to the midpoint X1 by the same amount of distance. For example, move both photogates 5 cm closer to the midpoint.
  8. 7. Repeat the data recording process. That is, measure and record the new distance "D" between the photogates in the Data Table. Place the cart on the track. Release the cart. Type in the distance. Click "Enter".
  9. Continue to decrease the distance between photogates by equal amounts. Make sure the photogates are equidistant from the midpoint X1. Record data as described above. (Stop when the photogates are 10 centimeters apart.)
  10. Click "Stop Sampling" to end data recording.

 

ANALYZING THE DATA

Trial Distance "D" (m)
1
2
3
4

  1. Click the "Statistics" button in the lower left corner of the Graph to open the Statistics area on the right side of the Graph.
  2. Click the "Statistics" menu button in the Statistics area of the Graph. Select "Curve Fit, Linear Fit" from the Statistics menu. The "a1" term of the linear equation in the Stats area is the Y-intercept of the best fit line for your data.

 

QUESTIONS

  1. Which of the average speeds that you measured do you think gives the closest approximation to the instantaneous speed of the cart as it moved through the midpoint X1 ?
  2. What is the relationship between the "Y-intercept" on the Graph and the instantaneous speed of the cart as it moved through the midpoint X1 ?
  3. What factors (accuracy of timing, object being timed, release of object, type of motion) influence the results? Discuss how each factor influences the result.
  4. Are there ways to measure instantaneous speed directly, or is instantaneous speed always a value that must be derived from average speed measurements?

© Frank L. H. Wolfs, University of Rochester, Rochester, NY 14627, USA

Last updated on Sunday, February 11, 2001 21:24