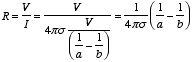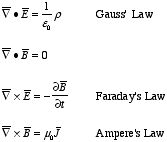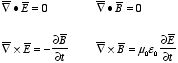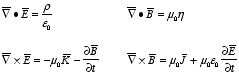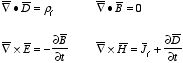Chapter 7. Electrodynamics
7.1. Electromotive Force
An electric current is flowing when the electric charges are in motion.
In order to sustain an electric current we have to apply a force on these
charges. In most materials the current density
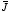
is proportional to the force per unit charge:
The constant of proportionality σ is called the
conductivity of the material. Instead of specifying the conductivity, it
is more common to specify the resistivity ρ:
For conductors the resistivity is typically 10-8 Ω-m; for
semiconductor it varies between 0.01 Ωm and 1 Ω-m, and for insulators
it varies between 105 Ω-m and 106 Ω-m. In most
cases the force on the charges is the electromagnetic force. In that case the
current density is equal to:
If the velocity of the charges is small the second term can be ignored, and
the equation for

reduces to
Ohm's Law:
Consider a wire of cross-sectional area A and length L. If a
potential difference V is applied between the ends of the wire, it will
produce an electric field inside the wire of magnitude
The current density in the wire is therefore equal to
The total current flowing through the wire is therefore equal to
This equation shows that the current flowing from one electrode to the
other electrode is proportional to the potential difference between them. This
is a rather surprising result since the charge carriers are constantly
accelerating. However, the proportionality between the current and the
potential difference has been found to be correct for most materials. This
relation can be written as
The constant of proportionality R is called the resistance of
the material. It is in general a function of the geometry of the system and the
conductivity of the materials between the electrodes. The unit of resistance is
the ohm (Ω). The resistance of the wire is equal to
To create a current we have to do work. The work required to move a unit
of charge across a potential difference V is equal to V. To
establish a current I, we need to deliver a power P
where
The unit of power is the Watt (1 W = 1 J/s). The work done by the
electric force on the charge carriers is converted into heat (Joule
heating).
Example: Problem 7.1
Two concentric metal
spherical shells, of radius a and b, respectively, are separated
by weakly conducting material of conductivity σ.
a) If they are
maintained at a potential difference V, what current flows from one to
the other?
b) What is the resistance between the shells?
a) Suppose a
charge Q is placed on the inner shell. The electric field in the region
between the shells will be equal to
The corresponding potential difference between the spheres is equal
to
Therefore, in order to maintain a potential difference V between the
spheres, we must place a charge Q equal to
on the center shell. The total current flowing between the two shells is
equal to
b) The resistance between the shells can be obtained from Ohm's
law:
Example: Problem 7.2
a) Two metal objects are embedded in
weakly conducting material of conductivity σ (see Figure 7.1). Show
that the resistance between them is related to the capacitance of the
arrangement by
b) Suppose you connected a battery between 1 and 2 and charged them up to a
potential difference V0. If you then disconnect the battery,
the charge will gradually leak off. Show that V(t) =
V0 exp(- t/τ), and find the time constant
τ in terms of ε0 and
σ.
a) Suppose a charge Q is placed on the positively
charged conductor. The current flowing from the positively charged conductor is
equal to
where the surface integral is taken over a surface that encloses the
positively charged conductor (for example, the dashed surface in Figure 7.1).
The expression for I can be rewritten in terms of the electric field
as

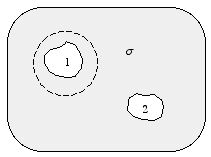 Figure
7.1. Problem 7.2.
Figure
7.1. Problem 7.2.Using Gauss's law to express the surface integral of

in terms of the total enclosed charge we obtain
The charge on the conductor is related to the capacitance of the
arrangement and the potential difference between the conductors:
The current I is therefore equal to
The resistance of the system can be calculated using Ohm's law:
b) The charge Q residing on the positively charged conductor is
equal to
This equation can be rewritten as
and has the following solution:
The potential difference V is equal to
The decay constant τ is equal to
In any electric circuit a current will only exist if a driving force is
available. The most common sources of the driving force are batteries and
generators. When a circuit is hooked up to a power source a current will start
to flow. In a single-loop circuit the current will be the same everywhere.
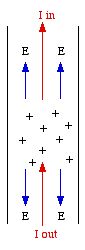
Consider the situation in which the currents are not the same (see Figure 7.2).
If Iin > Iout then positive
charge will accumulate in the middle. This accumulation of positive charge will
generate an electric field (see Figure 7.2) that slows down the incoming charges
and speeds up the outgoing charges. A reduction in the velocity of the incoming
charges will reduce the incoming current. An increase in the velocity of the
outgoing charges will increase the outgoing current. The current will change
until Iin = Iout.
The total
force f on the charge carriers (per unit charge) is equal to the sum of
the source force, fs, and the electric force:
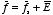
 Figure
7.2. Current flow.
Figure
7.2. Current flow.The work required to move one unit of charge once around the circuit is
equal to
where
ε is called the
electromotive force or
emf.
The emf determines the current flowing through the circuit. This can be most
easily seen bye rewriting the force

on the charge carriers in terms of the current density
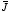
Here, a is the cross-sectional area of the wire (perpendicular to
the direction of the current).
Example: Problem 7.5
a) Show
that electrostatic force alone cannot be used to drive current around a
circuit.
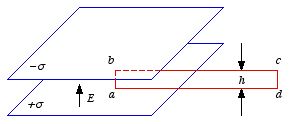
b) A rectangular loop of wire is situate so that one end is between
the plates of a parallel-plate capacitor (see Figure 7.3), oriented parallel to
the field E = σ/ε0. The other end is
way outside, where the field is essentially zero. If the width of the loop is
h and its total resistance is R, what current flows?
Explain.
 Figure
7.3. Problem 7.5.
Figure
7.3. Problem 7.5.a) If only electrostatic forces are present then the force per unit charge
is equal to the electrostatic force:
The associated emf is therefore equal to
for any electrostatic field.
b) The only force on the charge carriers in
the wire loop is the electric force. However, in part a) we concluded that the
emf associate with an electric force, generated by an electrostatic field, is
equal to zero. Therefore, the emf in the wire loop is equal to zero, and
consequently the current in the loop is also equal to zero. Note: at first
sight it might appear that there is a net emf, if we assume that the electric
field generated by the capacitor is that of an ideal capacitor (that is a
homogeneous field inside and no field outside). Under that assumption, the emf
is equal to
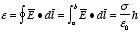
The contribution of the path integral from c to d is equal to
zero since the electric field is zero there, and the contribution of the path
integrals between b and c and between a and d is
equal to zero since the electric field and the displacement are perpendicular
there. Clearly the calculated emf is non-zero, and disagrees with the result of
part a). The disagreement is a result of our incorrect assumption that the
electric field outside the capacitor is equal to zero (there are fringing
fields).
An important source of emf is the generator. In these devices
the emf arises from the motion of a conducting wire through a magnetic field.
Consider the system shown in Figure 7.4 (note: the magnetic field is only
present in the region left of the dashed line). Consider the free charges on
the conductor. Since it is moving with a velocity v in a magnetic field
it will experience a magnetic force. The force on a positive charge q
located ion segment ab of the wire loop is equal to
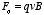
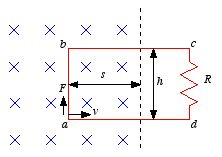 Figure
7.4. The generator.
Figure
7.4. The generator.The magnetic force per unit charge is therefore equal to
Since there are no other forces acting on the charges, the emf generated
will be entirely due to this magnetic force. The emf will be equal to
The magnetic flux intercepted by the wire loop is equal to
The rate of change of the magnetic flux is equal to
Comparing the rate of change of enclosed magnetic flux and the induced emf
we can conclude that
This relation is called the flux rule for motional
emf.
7.2. Faraday's Law
When a conducting wire moves in a constant magnetic field an emf is
generated equal to
In this case, the magnetic force is responsible for the emf. However, the
same emf is generated when the wire is stationary and the magnetic field is
moving. In this case, the magnetic force does not play a role (since v =
0) and an electric field is responsible for the emf. This electric field is not
an electrostatic field (since electrostatic fields can not generate an emf; see
Problem 7.5) but is induced by the changing magnetic field. The line integral
of this electric field is equal to
This equation can be rewritten by applying Stoke's theorem:
Since we have not made any assumption about the surface, this equation can
only be true if
This relation is called Faraday's law in differential form. The
direction of the currents generated by the changing magnetic field can be
obtained most easily using Lenz's law which states that
“ If
a current flows, it will be in such a direction that the magnetic field it
produces tends to counteract the change in flux that induced the emf.
“
Example: Problem 7.14
A long solenoid of radius
a, carrying N turns per unit length, is looped by a wire of
resistance R (see Figure 7.5).
a) If the current in the solenoid is
increasing,
what current flows in the loop, and which way (left or right) does it pass
through the resistor.
b) If the current I in the solenoid is constant
but the solenoid is pulled out of the loop and reinserted in the opposite
direction what total charge passes through the resistor?
 Figure
7.5. Problem 7.14.
Figure
7.5. Problem 7.14.a) Assume that the solenoid is an ideal solenoid; that is
If the current in the solenoid increases, the strength of the magnetic
field also increases. The rate of change in the strength of the magnetic field
is equal to
The magnetic flux intercepted by the wire loop is equal to
The corresponding rate of change of the magnetic flux is equal to
The induced emf can be obtained from the flux law:
The current induced in the wire loop is equal to
The solenoidal magnetic field points from left to right. An increase in
the strength of the magnetic field will induce a current in the loop directed
such that the magnetic field it produces point from right to left (Lenz's law).
Therefore, the current flows from left to right through the
resistor.
b) The change in the magnetic flux enclosed by the wire loop is
equal to
The current flowing through the resistor is equal to
This relation shows that
Substituting the expression for
we obtain
7.3. Inductance
Consider two loops: loop 1 and loop 2 (see Figure 7.6). A current
I1 flowing through loop 1 will produce a magnetic field at the
position of loop 2 equal to
The magnetic flux through loop 2 is equal to

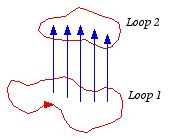 Figure
7.6. Inductance.
Figure
7.6. Inductance.Here, M21 is called the mutual inductance of the
two loops. It is a purely geometrical quantity that depends on the sizes,
shapes and relative positions of the two loops. It does not change if we switch
the role of loop 1 and loop 2: the flux through loop 2 when we run a current
I around loop 1 is exactly the same as the flux through loop 1 when we
send the same current I around loop 2.
Besides inducing an emf in a
nearby loop, the changing current in loop 1 also induces an emf in loop 1. The
flux through loop 1 generated by the current in loop 1 is equal to
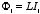
The constant of proportionality is called the self inductance. The
unit of inductance is the Henrie (H).
Example: Problem
7.19
A square loop of wire, of side s, lies midway between two
long wires, 3s apart and in the same place. (Actually, the long wires
are sides of a large rectangular loop, but the short ends are so far away that
they can be neglected.) A clockwise current I in the square loop is
gradually increasing: dI/dt = k = constant. Find the emf
induced in the big loop. Which way will the induced current flow?
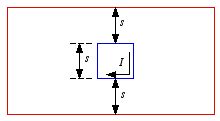 Figure
7.7. Problem 7.19.
Figure
7.7. Problem 7.19. The system is schematically shown in Figure 7.7. To calculate the emf
induces in the large loop we need to determine the mutual inductance M.
It is hard to calculate M in terms of a current in the square loop since
the magnetic field generated by this loop is rather complicated (and therefore
difficult to integrate). However, exploiting the equality of the mutual
inductances, we can also evaluate the inductance in terms of a current I
in the large loop. The magnetic field generated by the top wire of the large
loop is that of an infinitely long straight wire carrying a current I is
equal to
The flux associated with this magnetic field and intercepted by the square
loop is equal to
The magnetic field generated by the bottom wire at the position of the
square loop will be pointing in the same direction as the magnetic field
generated by the top wire at the position of the square loop. Since the square
loop is located at the same distance from the top wire as it is from the bottom
wire, the flux intercepted by the square loop is equal to
Therefore, the mutual inductance of the two loops is equal to
The induced emf in the large loop can now be calculated easily:

The direction of the flux through the large loop is pointing into the page.
This can be seen most easily by considering the magnetic field lines. Inside
the square loop, the field lines point into the page (right-hand rule). Since
the field lines form closed loops, they must be pointing out of the page
anywhere outside the square loop. However, the large wire loop only covers a
limited fraction of space, and therefore definitely will not intercept all field
lines outside the square loop. Therefore, there will be more field lines
pointing into the page then there are field lines pointing out of the page.
Consequently, the net magnetic flux will be pointing into the page. When the
current in the square loop increases the flux intercepted by the large loop will
increase. The induced emf will produce a magnetic field that counteracts this
increase in flux, and therefore produces a flux pointing out of the paper. The
right-hand rule shows that the direction of the current induced in the large
loop must be flowing in a counter-clockwise direction.
7.4. The Maxwell Equations
The electric and magnetic fields in electrostatics and magnetostatics are
described by the following four equations:
In systems with non-steady currents not all of these equations are valid
anymore. For example,
for every vector function. However, according to Ampere's law
which is only zero for steady currents (for which
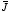
is a constant, independent of position). For non-steady currents
We thus conclude that Ampere's law does not hold for non-steady currents.
The failure of Ampere's law can also be observed in a system in which a
capacitor is being charged (see Figure 7.8). During the charging process a
current
I is flowing through the wire, and consequently there will be a
magnetic field present. The magnetic field generated by the charging current
can be calculated using Ampere's law. When we are far away from the capacitor
the generated magnetic field will be that of a line current. Consider an
Amperian loop of radius
r, centered on the wire. The line integral of
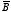
around this loop is equal to
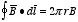
According to Ampere's law the line integral of
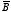
around a closed loop is proportional to the current intercepted by a surface
spanned by this loop. For the system shown in Figure 7.8 the intercepted
current is ill defined. Consider first surface 1. The current intercepted by
surface 1 is equal to
I. Surface 2 is also spanned by the Amperian loop,
but the current intercepted by this loop is zero. We thus conclude that
Ampere's law does not apply in systems where the current is not
continuous.
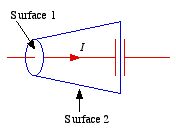 Figure
7.8. Charging a capacitor.
Figure
7.8. Charging a capacitor. Maxwell modified Ampere's law in the following manner:
The term added by Maxwell is called the displacement current. It is
defined as
Consider the region between the capacitor plates in Figure 7.8. The
electric field in this region is equal to
where we have assumed that the field produced is that of an ideal capacitor
with surface area A and the z axis is in the direction of the
current. The rate of change of the electric field is equal to
The surface integral of
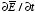
across surface 2 is therefore equal to
The surface integral of
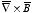
across surface 2 is equal to
The modification of Ampere's law by Maxwell insures that the surface
integral of
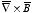
is independent of the surface chosen. In electrostatics and magnetostatics the
electric and magnetic fields are constant in time, and therefore, the new form
of Ampere's law reduces to the form of Ampere's law we have been using so
far.
In a region where there are no free charges or free currents Maxwell's
equations become very symmetric
The symmetry is broken when electric charges are present, unless besides
electric charges there are
magnetic monopoles. If the magnetic charge
density is equal to
η and the magnetic current is equal to
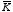
then Maxwell's equation become
To obtain Maxwell's equations that describe the electric and magnetic
fields in matter we must take the bound charges and bound currents into
account:
In the non-static case, the polarization can be time dependent. Therefore,
also the bound charge density is time dependent, and a net current can be
associated with the change in the bound charge density. This current is called
the
polarization current
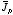
and is equal to
Maxwell's equations in matter are therefore equal to
It is common to rewrite Maxwell's equations in terms of the parameters we
can control (the free charge density and the free current density). Gauss's law
can be rewritten as
where
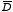
is called the
electric displacement. Ampere's law can be rewritten
as
where
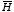
is called the
H field. The most general form of Maxwell's
equations, in terms of the free charges and free currents, is given by