Home Work Set # 3, Physics 217, Due: September 26,
2001
Problem 1
a) Twelve equal charges, q, are situated at the corners of a regular
12-sided polygon (for instance, one on each numeral of a clock face). What is
the net force on a test charge Q at the center?
b) Suppose one of the
12 q's is removed (the one at "6 o'clock"). What is the force on
Q?
c) Now 13 equal charges, q, are placed at the corners of a
regular 13-sided polygon. What is the force on a test charge Q at the
center?
d) If one of the 13 q's is removed, what is the force on
Q?
Problem 2
Find the electric field a distance z above the center of a square
loop (side s) carrying a uniform line charge λ (see Figure
1).
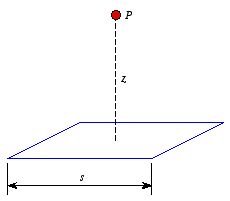 Figure
1. Problem 2.
Figure
1. Problem 2.
Problem 3
Find the electric field a distance z above the center of a flat
circular disc of radius R (see Figure 2), which carries a uniform surface
charge σ. What does your formula give in the limit R →
∞. Also check the case z » R.
 Figure
2. Problem 3.
Figure
2. Problem 3.
Problem 4
Suppose the electric field in some region is found to be
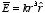
.
a) Find
the charge density
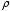
.
b) Find
the total charge contained in a sphere of radius
R, centered at the
origin. Do the calculation in two different ways.
Problem 5
Use Gauss's law to find the electric field inside and outside a spherical
shell of radius R, which carries a uniform surface charge density
σ.
Problem 6
A hollow spherical shell carries a charge density
in the region
a ≤
r ≤
b (see Figure 3).
a) Find the electric field in the region
r <
a.
b) Find
the electric field in the region
a <
r <
b.
c) Find the electric field in the region
b <
r.
d) Plot
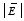
as function of
r.
 Figure
3. Problem 6.
Figure
3. Problem 6.
Problem 7
An infinite plane slab, of thickness 2
d, carries a uniform volume
charge density
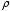
(see Figure 4). Find the electric field, as a function of
y, the
distance from the center. Plot
E versus
y, calling
E
positive when it points in the +
y direction and negative when it points
in the -
y direction.
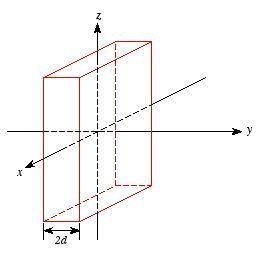 Figure
4. Problem 7.
Figure
4. Problem 7.



