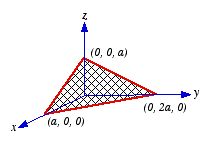
using the triangular surface shown in Figure 1. Express all your answers
in terms of a.
Problem 2 (30 points)A spherical shell of radius
R has a uniform surface charge density. The total charge on the shell is
q. Find the electrostatic energy of this system in two different ways.
Express all your answers in terms of
q and
R.
Problem 3 (35 points)Consider the following
three vector
functions:
(1)
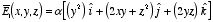
(2)
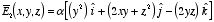
(3)
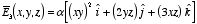
where
α is a constant with the appropriate units.
a) Which of these
three vector functions can describe an electrostatic field?
b) For the vector
function of part a) that can describe an electrostatic field find the
corresponding electrostatic potential at point
P(
x,
y,
z), using the origin as your reference point.
c) Find the charge
distribution that produces the electrostatic potential obtained in part
b).
Express all your answers in terms of
α,
x,
y,
and
z.